The stability limit of the self-excited chatter vibration in plunge grinding can be obtained by substituting s = jrn into the characteristic equations based on Figure 8.7 and Figure 8.8. Influence of the depth of cut, the workpiece speed, and the grinding width on the stability limit is illustrated in Figure 8.11 [Inasaki 1977a] for chatter vibration of both types: the regenerative effect on the workpiece surface and the regenerative effect on the grinding wheel surface. With respect to the former type of chatter vibration, absolute stability can be attained when the workpiece velocity is sufficiently low. On the other hand, the latter type of chatter vibration has a large area of instability, that is, most practical grinding conditions exist in the unstable region. Therefore, as far as the chatter vibration caused by the regenerative effect on the grinding wheel surface is concerned, it is necessary to know the increase speed of the vibration amplitude.
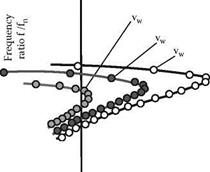
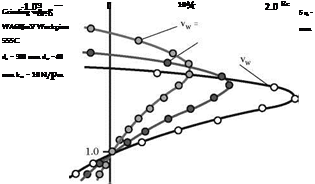
The positive real part of the roots of the characteristic equation is the index for the rate of increase of the vibration amplitude, while the imaginary part indicates the chatter frequency. Some calculated examples of the roots distribution are shown in Figure 8.12 and Figure 8.13 [Inasaki 1977a]. The important results deduced from those figures are [2]
Grinding wheel:
![]()
![]()
![]()
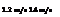
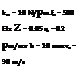
 WA60JmV
WA60JmV
Workpiece:
S55C
ds = 300 mm dw = 40 mm
![]()
 l_
l_
-1.0
a
FIGURE 8.12 Roots of workpiece regenerative chatter.
Figure 8.14 shows the calculated results of the positive real parts for the grinding wheel regenerative chatter. It is assumed here that the instability occurs at the frequency that gives the maximum positive real part. From this result, the following conclusions are deduced:
 |
• The development of chatter vibration becomes faster with larger depth of cut, larger grinding width, lower workpiece speed, and higher grinding wheel speed. With respect to the effect of depth of cut, however, it is necessary from the practical point of view to consider the increase of vibration amplitude against the amount of cumulative material removed. The calculated result shows that a larger amount of material can be removed before the grinding wheel comes to the end of its redress life with larger depth of cut.
= var.; ae = 1.0 pm/rev; 10 mm; vw = 0.3 m/s
![]()
![]()
![]()
![]()
![]()
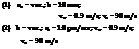
![]()

![]()

![]() = var.; ae = 1.0 pm/rev; 10 mm; vs = 30 m/s
= var.; ae = 1.0 pm/rev; 10 mm; vs = 30 m/s
• The rate of increase in vibration amplitude decreases with a decrease in the contact stiffness and increase in the wear stiffness of the grinding wheel. This result means that the influence of the grinding wheel hardness is complex. An increase of stiffness and damping in the mechanical system reduces the rate of chatter development.
A similar analysis can be conducted for internal as well as surface grinding [Inasaki 1977a]. The stability analysis of traverse grinding is much more complex than that of the plunge grinding. However, some theoretical and experimental investigations have been conducted for the workpiece regenerative chatter in cylindrical grinding [Shimuzu et al. 1978]. Important conclusions were
• The process tends to be unstable under the condition of lower traverse speed, higher workpiece speed, larger grinding wheel width, and smaller depth of cut.
• Chatter frequency increases with increases of traverse speed, grinding wheel width, depth of cut, and workpiece speed.
